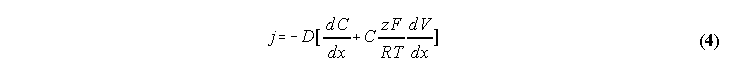The Nerve Impulse.
(C) F. Bezanilla, 1998-2020
TABLE
OF CONTENTS
INTRODUCTION
Axons are responsible for the transmission of information between different
points of the nervous system and their function is analogous to the wires that
connect different points in an electric circuit. However, this analogy cannot
be pushed very far. In an electrical circuit the wire maintains both ends at
the same electrical potential when it is a perfect conductor or it allows the
passage of an electron current when it has electrical resistance. As we will
see in these lectures, the axon, as it is part of a cell, separates its internal
medium from the external medium with the plasma membrane and the signal conducted
along the axon is a transient potential difference(1)
that appears across this membrane. This potential difference, or membrane
potential, is the result of ionic gradients due to ionic concentration
differences across the membrane and it is modified by ionic flow that produces
ionic currents perpendicular to the membrane. These ionic currents give rise
in turn to longitudinal currents closing local ionic current circuits that allow
the regeneration of the membrane potential changes in a different region of
the axon. This process is a true propagation instead of the conduction phenomenon
occurring in wires. To understand this propagation we will study the electrical
properties of axons, which include a description of the electrical properties
of the membrane and how this membrane works in the cylindrical geometry of the
axon.
Much of our understanding of the ionic mechanisms responsible for the initiation
and propagation of the action potential (AP) comes from studies on the squid
giant axon by A. L. Hodgkin and A. F. Huxley in 1952. The giant axon has a diameter
in excess of 0.5 mm, allowing the introduction of electrodes and change of solutions
in the internal medium. These studies have general relevance because the properties
of the squid axon are very similar to non-myelinated nerves in other invertebrates
and vertebrates, including man.
The Capacitance and Resistance of the membrane.
The plasma membrane is made of a molecular lipid bilayer. Inserted in this
bilayer, there are membrane proteins that have the important function of transporting
materials across the membrane. The lipid bilayer acts like an insulator separating
two conducting media: the external medium of the axon and the internal medium
or axoplasm. This geometry constitutes an electric capacitor(2)
where the two conducting plates are the ionic media and the membrane is the
dielectric. The capacitance c of a capacitor increases with the area
of the plates and decreases with the separation between the plates according
to the the relation

where A is the membrane area, d is the separation between
the plates or the membrane thickness and is the dielectric constant. In the
case of the membrane, it is more convenient to define the capacitance as independent
of the amount of area involved and call it the specific capacitance Cm
which is defined as the capacitance per unit area or c/A. Replacing
this definition in eq (1) we find

As the thickness d is only 25 A, the specific capacitance of the membrane
is very high, close to 1 µF/cm2. Having the properties of a capacitor,
the membrane is able to separate electric charge, achieved by a difference
in the number of anions and cations on each sideof the membrane; this charge
separation, in turn produces a potential difference across the membrane. In
a capacitor the potential difference V is related to the charge Q
by

where C is the capacitance. It is important to notice that in the case
of the plasma membrane a small amount of charge separation is able to generate
a large potential difference. For example, to obtain a membrane potential of
100 mV it is necessary to separate the product of Cm
=1(µF/cm2) times Vm = 0.1
(Volts), that is, Q=0.1 µCoulombs/cm2.To get an idea of
the magnitude of this charge, we can compute the number p of monovalent
ions that must be separated across the membrane to explain this charge
p = 0.1 x 10-6 (coul/sq cm)/1.6 x 10-19
(coul/ion)
= 6.25 x 1011 (ion/cm2)
which corresponds to only 6250 ions per µm2 of membrane.
The energy to put an ion into the lipid bilayer is so large that
we would expect the membrane to be practically impermeable to ions. However,
experimentally, it has been found that the membrane presents a finite permeability
to cations and anions. Today we know that this permeability is mediated through
specialized proteins that can act as carriers or channels for the passage of
charged species. The detail of the operation of channels will be described later
in this course. What is relevant here is the fact that ions can penetrate through
specialized pathways which constitute the membrane electrical conductance
(conductance is the reciprocal of resistance). This conductance will be another
element of our electric circuit that will represent the electrical characteristics
of the membrane.

Again we will define units that are independent of the membrane
area. If we measure the electrical resistance of a membrane of 1 sq cm of surface
area, it will be 10 times larger than the resistance of a membrane with the
same characteristics but of 10 sq cm surface area. This is because the resistance
decreases as the access area increases. For this reason, we can define the membrane
specific resistance Rm in units of
ohms sq cm or specific conductance Gm
in units of Siemens/cm2 (S/cm2).
The Membrane Potential.
How is it possible to separate charge across the membrane? Let us take a simple
example. Assume that we have a membrane separating two compartments (Fig. 1)
that has channels that are only permeable to potassium and no other ions can
permeate. Initially the channels are closed and we add 100 mM of KCl to the
lower compartment (say the interior of the cell) and 10 mM of KCl to the upper
compartment (the outside). As we have added a neutral salt, there will be the
same number of cations than anions in the lower compartment and the same will
be true for the upper compartment (even though the total number of ions is 10
times lower in the upper compartment).The consequence of the electroneutrality
in each side will be zero charge difference across the membrane and consequently
the membrane potential difference will be zero. This is because from eq. (3)
we can write that V=Q/C, where V is the potential difference
and Q is the excess charge. The permanent thermal motion of the ions
will make them move randomly but they will not be able to cross the membrane
because they are poorly permeant through the bilayer and the channels are closed.
Suppose that at one point we open the channels. Then, as there are 10 times
more K+ ions in the bottom compartment than in the top compartment,
there will be 10 times more chances of an ion crossing up than down. This initial
situation is schematically pictured in Fig. 1A where a K+ ion (the
black balls) is crossing the channel in the upward direction leaving a Cl-
ion behind. This flow, which is proportional to the concentration gradient,
increases the top compartment by one positive charge and the lower compartment
by one negative charge, producing a charge separation (Fig. 1B). This charge
separation introduces a non-random new electrostatic force acting on the ions,
as pictured in the box insets of Fig. 1. This electrostatic force tends to drive
the ions from the top compartment into the bottom compartment and at the same
time it tends to brake the flow in the opposite direction. The final result
is that the charge separation will build up a voltage across the membrane (V=Q/C)
that will continue to increase until the flow in both directions becomes equal
due to the increased electrostatic force that will tend to balance the flow
produced by the concentration gradient. When that happens, any ion that crosses
in one direction will be counterbalanced by another crossing in the opposite
direction, maintaining an equilibrium situation. This potential difference is
then called the equilibrium potential or Nernst potential.
The above discussion can be put in more quantitative terms by expressing the
net ion flow j in terms of the chemical and electrical gradients:
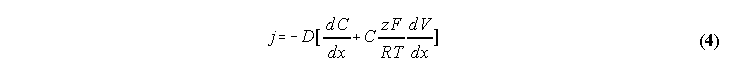
where D is the diffusion coefficient, C is the concentration,
R is the gas constant, V is the voltage, z is the
valence, F is the Faraday constant, and T is the temperature.
When j=0 (no net flow), eq (4) can be integrated and we get:

which is the Nernst equation that relates the voltage V across the
membrane which is in equilibrium with the concentration gradient established
by the concentrations Co, outside, and Ci,
inside. It is customary to call this voltage the equilibrium potential of the
ion "N" Ee, and by calling
the external and internal concentrations of the ion "N" No
and Ni, respectively we can rewrite eq. (5)
as follows:

More than one specific channel.
In the nerve membrane there are several types of channels, each of which is
selective to an specific ion, such as Na+ or K+. Therefore
the situation of zero net flow across the membrane does not depend on one particular
ion concentration gradient but it involves the concentration of the other permeant
ions and their relative permeabilities. In this situation we have to consider
the individual fluxes jNa, jK, etc.
and the solution when the sum of all the flows is zero gives the Goldman-Hodgkin-Katz
equation which can be written as

where the permeabilities for the ion k is written as Pk
and the concentrations of the ion are given by its chemical symbol followed
by the subindex indicating the side of the membrane, with i for inside
and o for outside. Thus, according to this equation, the voltage across
the membrane is determined by the concentrations of all the ions and is most
affected by the ion with the highest permeability. If E, as computed
from eq. (7) is equal to the E of the Nernst equation (eq. 6) for one
particular ion, we say that that ion is in equilibrium.
Real channels are not perfectly selective. The selectivity
of ion channels is not perfect and, for example in K+ channels, for
every 20 K+ ions that flow through the channel, one Na+
ion can get through. This means that we cannot apply the Nernst equation to
compute the potential that produces zero flow across the channel because more
than one ion is involved. Instead, we could use the Goldman-Hodgkin-Katz equation
(with PK/PNa=20, in the case of the K channel) and the potential
predicted by the equation would be called reversal potential, instead
of equilibrium potential, at which the net flow of charge through the channel
is zero. .

The electric Model of the Membrane
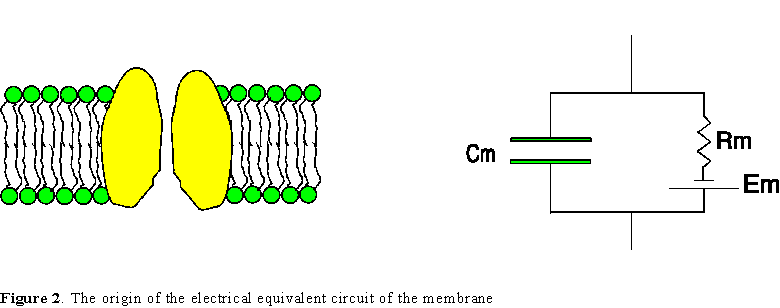
We have now described the capacitance of the membrane, mainly
given by the bilayer, the resistance of the membrane given by the ionic channels,
and we must now include the membrane potential. As explained in the
previous paragraphs, this membrane potential exists even in the absence of stimulus
or external electric field due to the charge separation produced by the ion
redistribution under the influence of chemical and electrical gradients. In
resting conditions, this voltage is called the resting potential and
it can be represented as a battery that must be in serieswith the membrane resistance
(Fig. 2). This battery of electromotive force (3)Em and the membrane resistance Rm
may be considered as the equivalent electric circuit of the membrane of the
axon, which includes all the membrane resistances and batteries of the different
systems of ionic channels each one with its own conductance gi
(where gi =1/Ri)
and reversal potential Ei. Notice
that the battery is in series with the resistance which implies that
the membrane potential is equal to Em
only when there is no current flow through the resistance Rm,
that is, in open circuit conditions, or when it is measured electrometrically
(without draining current through the membrane).

The circuit in Fig. 2 is a minimal representation of the membrane. It is important
to note that this representation refers to a membrane element of very small
dimensions, such that the potential could be considered constant along each
side of the membrane. This is only true if the element is made infinitesimally
small bacause any finite size may not be isopotential due to the electrical
resistance of the medium and the geometry of the axon. As we will see below,
in the case of the axon we will have to locate this basic circuit of the membrane
element in the cylindrical geometry of the axon which will make the final circuit
quite complicated. But before incorporating the geometry, we can study the basic
properties of our elemental circuit which will be extremely useful in understanding
the electrical behavior of the elementary unit that constitutes the axonal membrane.

Basic current equation through the conductive
pathways:
The current through the conductive part of the membrane can be expressed as
a product of a conductance and a driving force:

where V-Ee is the driving force and
g is the conductance (reciprocal value of resistance, R=1/g) expressed
in Siemens( S=1/ohm). V is the membrane potential and Ee
is the reversal potential for that pathway. If the pathway is selective to only
one ion species, Ee corresponds to the equilibrium potential
of that species e (the potential predicted by the Nernst equation).
There are several types of conductance and their classification is done according
to the type of channel involved. Thus, we have: Sodium, selective to Na; Potassium,
selective to K; Chloride, selective to Cl; Calcium, selective to Ca, etc. Currents
through each conductance type: INa, IK,ICl, etc
In the resting membrane, the resting potential V
is constant and there is no net current crossing in or out of the cell,
although each individual conductance may be carrying a net current, therefore:

Replacing the current expression for each conductance, from eq (8) into eq (9):

Solving for V in eq (10) we get:


This expression is similar to the Goldman-Hodgkin-Katz equation but it considers
conductances and reversal potentials instead of permeabilities and concentrations.
In several excitable membranes, such as the squid axon, gCl
is small but there are other type of conductances that have been lumped together
under the name of leakage or gL, which
is then substituted for gCl.
When simulating the membrane potential using this link, move the slider with
the mouse to modify the conductances.


In this section, we will study the currents through the different ionic pathways
and the resulting voltage across the membrane. The conventions will be that
the inside of the membrane faces down and all the voltages will be referred
to the outside. For example, if the membrane potential is -80 mV it means that
the inside is negative with respect to the outside. The variable conductances
(or variable resistances) are represented by a box with a side cursor (or arm)
that can slide up and down the side of the box (see Fig. 3). Thus, when the
cursor is close to the top the resistance between the two ends is minimum and
becomes maximum when the arm is close to the bottom (Fig. 3). The equilibrium
(or reversal) potentials are represented by batteries with the value written
near the battery symbol. In addition, the intensity of the current is represented
by the degree of shading of the wires, being black the highest intensity and
white zero current. The arrows indicate the direction of positive ions current
flow.
With these conventions, we can study the influence of the conductances and
reversal potentials in the final membrane potential in the steady state.
First Case. Only one conductance is present (Figs 4A and 4B).
All the other conductances are zero (that is, resistances are infinite) represented
by an interruption in the connection of the variable resistor. This means that
there is no current circulation in any of the branches with infinite resistance.
This is represented in Fig. 4A for the case of finite K conductance and all
the others zero. Notice that the membrane potential will be equal to the K equilibrium
potential (in this case -73.3 mV) because there is no current circulation through
any other resistor and, since we are considering steady state, the capacitor
does not drain any current (we will study later the origin of capacitive current).
In Figure 4B there is a representation of the case when only the Na conductance
is present and all the others are zero. As expected, in this case the membrane
potential will be identical to the Na reversal potential, in this case +41.3
mV. The situation depicted in Fig. 4 is a rough approximation of what occurs
during an action potential: at resting the membrane is selective to K and the
membrane potential is near -73 mV and during the action potential the membrane
becomes selective to Na and the membrane potential reverses and becomes positive.


Second Case (Figs. 5 and 6). Now we consider the more realistic
situation where all the conductances have a finite value, although different
among them. In the resting condition, the predominant conductance is potassium
as shown in Fig. 5. In the resting condition all the conductances are small
but the K conductance is larger than all the others. Notice that the relative
values of the conductances (in mS/sq cm) are gK=0.37, gNa=0.02
and gL=0.3. The K battery will drive an outward current
through the K conductance that will be drained by the leakage and Na branches
to make the total current equal zero. The resulting membrane potential will
be between the K reversal potential and Na reversal potential, but closer to
the EK because gK
is the largest conductance. Finally, let us consider the case when the Na conductance
predominates as during the rising phase of the action potential. In this case,
the actual sodium conductance increases several fold over the resting condition,
becoming the dominant conductance. This produces a large flow of inward current
through the Na conductance that is drained out by the K and leakage conductances
(see Fig. 6).
The action potential is the result of changing the membrane from a K selective
condition to a Na selective condition. We must explain now how this occurs.
To understand how these conductances shifts occur, we must study their ability
to change with the membrane voltage, or their "voltage dependence".This
leads to a description of voltage dependent conductances. In voltage dependent
conductances the value of the conductance depends on the membrane potential.
VOLTAGE DEPENDENT
CHANNELS
Back to Contents
NOTES:
1. A potential difference exists between
two points whenever the introduction of a conducting path between the two points
would result in spontaneous charge transfer or electric current.
2. An electric capacitor is a device that
allows the separation of electric charge on two conducting plates by a material,
or dielectric, that is not able to sustain an electric current.
3. The electromotive force of a battery is the voltage measured
from the battery in the absence of current circulation